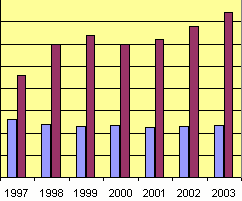

A police car cruises on a highway at 110 km/h. The other cars drive with either 80, 100, 120, 140, or 160 km/h. The policemen measure the speed of every car they pass and of every car passing them. After a while, they observe that 20% of the cars measured has speed of 160km/h, and 20% of them has a speed of 140km/h (just wait a little while, you will see that the observed frequencies are around these values after a while.
What can be said about the frequencies of these speeds among all cars on the road this day?
You can change the police car speed in the first text field, please choose a multiple of 10 between 80 and 160. You can change the probabilities of the cars driving with 80, 100, 120, 140, or 160 km/h in the next five text fields. Below, the frequency distribution of these car speeds is given. On the right of the applet, under the heading "observed distribution", the numbers of percentages of cars of the different speeds observed by the police car a given. Note that the police car only observes a car if it vertically on the same line than the police car.
Discuss this model, make some experiments, also try to get a theoretical formula how the probability distribution of speeds (left) and the observed frequency distribution of the speeds (right), given a certain police car speed. If you have more time and some background in Calculus, you can also discuss the case of continuous probability distributions.
Erich Prisner, May 2006