- Consider the following game:
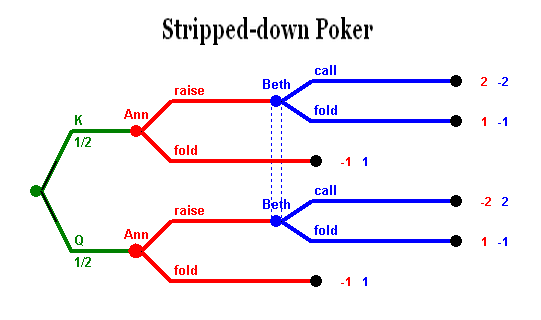
Both Ann and Beth put one dollar in the pot.
Ann gets a card from a stack of 4 Queens and 4 Kings and looks at it privately.
Then Ann either folds, in which case Beth gets the money in the pot, or raises.
Raising means that Ann has to put another dollar in the pot. When Ann has raised,
Beth either folds, in which case Ann gets the pot, or Beth calls by putting also
one more dollar in the pot. If Beth calls, Ann gets the pot if she has a King,
otherwise Beth gets the pot.
- Draw the Extensive Form of the game. How many pure strategies does Ann have,
and how many pure strategies does Beth have?
- Draw the Normal Form of the game.
- Analyze the game. How should Ann and Beth play?
- Consider the following game:
Both Ann and Beth put one dollar in the pot. Ann gets a card and looks at it privately.
Then Ann either checks, in which case Ann gets the money in the pot if Ann's card is red,
or Beth gets the pot if Ann's card is black. Ann can also raise by putting another dollar in the pot.
Now Beth either folds, in which case Ann gets the pot, or Beth calls by putting one more
dollar in the pot. If Beth calls, Ann gets the pot if she has a red card, otherwise Beth gets the pot.
- Draw the Extensive Form of the game. How many pure strategies does Ann have,
and how many pure strategies does Beth have?
- Draw the Normal Form of the game.
- Analyze the game. How should Ann and Beth play?
- Consider the following game:
Ann starts the game by selecting (Ann doesn't draw, she chooses) two cards from a deck of cards containing
four Queens and four Kings. Ann puts these cards face down in front of Beth. Beth is allowed
to see one of them. Then Beth must guess whether the two
cards are two Kings, two Queens, or one King and a Queen. If she is right, she
wins $1 from Ann, otherwise she has to pay $1 to Ann.
- Draw the Extensive Form of the game. How many pure strategies does Ann have,
and how many pure strategies does Beth have?
- Draw the Normal Form of the game.
- Analyze the game. How should Ann and Beth play?
- Consider the following game:
Ann starts the game by selecting (Ann doesn't draw, she chooses) one card from a deck of cards containing
four Queens and four Kings. Ann puts this cards face down in front of Beth. Beth is not allowed to see it,
but is allowed to see one card of the remaining deck of seven cards. Then Beth must guess whether the
card face down in fron of her is a King or a Queen. If she is right, she
wins $1 from Ann, otherwise she has to pay Ann $1.
- Draw the Extensive Form of the game. How many pure strategies does Ann have,
and how many pure strategies does Beth have?
- Draw the Normal Form of the game.
- Analyze the game. How should Ann and Beth play?
- Consider the following game:
Ann starts the game by selecting (Ann doesn't draw, she chooses) two cards from a deck of card containing
four Queens and four Kings. Ann puts these cards face down in front of Beth. Beth is not allowed to see them,
but is allowed to see one card of the remaining deck. Then Beth must guess whether the two
cards are two Kings, two Queens, or one King and a Queen. If she is right, she
wins $1 from Ann, otherwise she has to pay Ann $1.
- Draw the Extensive Form of the game. How many pure strategies does Ann have,
and how many pure strategies does Beth have?
- Draw the Normal Form of the game.
- Analyze the game. How should Ann and Beth play?
Consider the following game:
KUHNPOKER: We play with a 8 cards deck, four "1"s and four "2"s.
Every player gets one card and looks at it secretly.
The start bet is $2.
Ann moves first by either checking or raising.
- If Ann checks, then Beth can check or raise.
- If Beth checks, both cards are revealed and the player with the higher card wins the pot of $4.
splitting it again equally in case of a draw.
- If Beth raises, she increases the bet to $3. Then Ann has two options,
she can either fold or call.
- If Ann folds, Beth gets the pot money of $5, i.e. wins $2.
Ann's card is not revealed in that case.
- If Ann calls, she also increases her bet to $3. Then both cards are revealed again,
and the player with the higher card gets the money of $6, i.e. wins $3. Again, in case of a draw
the money is split equally.
- If Ann raises, she increases the bet to $3. Then Beth has two options,
she can either fold or call.
- If Beth folds, Ann gets the pot money of $5, i.e. wins $2.
Beth's card is not revealed in that case.
- If Beth calls, she also increases her bet to $6. Then both cards are revealed again,
and the player with the higher card gets the money of $6, i.e. wins $3. Again, in case of a draw
the money is split evenly.
- Draw the Extensive Form of the game. How many pure strategies does Ann have,
and how many pure strategies does Beth have?
- Draw the Normal Form of the game.
- Analyze the game. How should Ann and Beth play?
- The following is cited from the von Neumann-Morgenstern monograph
and analyzes a situation discussed in
[Conan Doyle, The Adventures of Sherlock Holmes, New York 1938, 550-551]:
"Sherlock Holmes desires to proceed from London to Dover and hence to the
Continent in order to escape from Professor Moriarty who pursues him.
Having boarded the train he observes, as the train pulls out, the appearance
of Professor Moriarty on the platform. Sherlock Holmes takes it for granted---and
in this he is assumed to be fully justified---that his adversary, who has seen him,
might secure a special train and overtake him. Sherlock Holmes is faced with the alternative of
going Dover or leaving the train at Canterbury, the only intermediate station.
His adversary---whose intelligence is assumed to be fully adequate to visualize these
possibilities---has the same choice. Both opponents must choose the place of their
detrainment in ignorance of the other's corresponding decision. If, as a result of
these measures, they should find themselves, in fine, on the same platform,
Sherlock Holmes may with certainty expect to be killed by Moriarty. If Sherlock
Holmes reaches Dover unharmed he can make good his escape."
a) Formulate this situation as a zero-sum game, where Professor Moriarty killing
Sherlock Holmes counts as -100 for Sherlock Holmes, Holmes leaving at Dover and
Moriarty stopping at Canterbury counts as 50 for Holmes, and finally Holmes leaving
at Canterbury while Moriarty leaves at Dover counts as a draw, 0 for Holmes,
since then he still has to find a way to escape.
b) Analyze the game with the methods described above.
- It is known that two smugglers will use either airport or seaport
on Saturday, trying to smuggle 1000 grams of plutonium. The two smuggler will
have the plutonium under their clothes, either both 500 grams, or one 1000 grams
and the other nothing. Two policeman wait for them, either on airport or on seaport.
If there are at least as many policemen at a location than smugglers, all this smugglers at that location
get caught. If on one
location no police waits, all smuggled material at that location gets through.
Finally,
if only one policeman waits on one location, facing two smuggler there,
then he will catch one of them (and the material he carries) with 50% probability,
in the other 50% of the cases the two smugglers can trick the policeman and smuggle
everything without getting caught.
Both parties, police and smugglers,
only care about the amount of material caught. How should they act?
(compare [compare Davis, page 25])
- You have two valuables: A $900,000 diamond ring in your bedroom, and a $100,000
porsche in your garage. When the alarm rings in the night, you can only guard one of them---ring or
porsche. If the burglar finds any of them unguarded, he just takes it. But if the burglar
tries to steel any of them and you are there, hidden, you catch the burglar, and the burglar gets
1 month of jail, worth -$1000 for him. What will you do, and what will the burglar do?
(compare [Davis, page 26])
- A convict has two possible escape routes: The main highway or the forest.
The sheriff can only cover one of these possibilities.
If both choose differently, the convict escapes.
If both choose the highway, the convict will be caught.
If both choose the forest, the chance of escape is 2/3.
What will both do? (compare [Davis, page 41])
- Analyze STRIPPED-DOWN POKER, described by this extensive form:
How would Ann and Beth play optimally, and how much can they expect if they play optimally?
- Analyze MYERSON POKER, described by this extensive form:
How would Ann and Beth play optimally, and how much can they expect if they play optimally?
- Analyze the variant of MYERSON POKER that is played with
4 Queen and only 3 King cards.
How would Ann and Beth play optimally, and how much can they expect if they play optimally?
- Analyze the variant of STRIPPED-DOWN POKER that is played with
4 Queen and only 3 King cards.
How would Ann and Beth play optimally, and how much can they expect if they play optimally?
- How many Queens and Kings should be used in MYERSON POKER to make it a fair game?
- How many Queens and Kings should be used in STRIPPED-DOWN POKER to make it a fair game?
